Trigonometric Identities and Formula: Check Complete List

Trigonometric Identities and Formula are essential equations in trigonometry that establish relationships between different trigonometric functions. Learn key types and applications in solving problems.

Trigonometric Identities are fundamental equations in mathematics that relate the six trigonometric functions sine, cosine, tangent, cosecant, secant, and cotangent to each other.

These identities are essential tools used to simplify trigonometric expressions, solve equations, and prove theorems in geometry, calculus, and physics.

They are derived from the relationships between the angles and sides of a right-angled triangle or the unit circle. Some of the key types of trigonometric identities include reciprocal identities, Pythagorean identities, sum and difference identities, double and half-angle identities, and product-sum identities.

Mastering these identities is crucial for anyone studying advanced mathematics, as they allow for the manipulation of complex trigonometric expressions and facilitate problem solving in various scientific fields.

Trigonometric Identities Define

Trigonometric identities are mathematical equalities that involve trigonometric functions and are valid for all values of the variables in the equation.

These identities are specifically applicable to right-angled triangles and relate to the side lengths as well as the angles within the triangle. The foundation of all trigonometric identities lies in the six trigonometric ratios: sine, cosine, tangent, cosecant, secant, and cotangent.

These ratios are defined using the sides of a right triangle, which include the adjacent side, opposite side, and hypotenuse. All the fundamental trigonometric identities are derived from these six key ratios.

Trigonometric Identities and Formula

1. Pythagorean Trigonometric Identities

Pythagorean Trigonometric Identities are derived from the Pythagorean theorem and relate the squares of the trigonometric functions of an angle in a right-angled triangle. These identities are essential in simplifying expressions involving trigonometric functions. The three primary Pythagorean identities are:

sin²(θ) + cos²(θ) = 1
This identity expresses the relationship between the sine and cosine functions. It is one of the fundamental identities and is derived directly from the Pythagorean theorem, where the sum of the squares of the opposite and adjacent sides of a right triangle equals the square of the hypotenuse.
1 + tan²(θ) = sec²(θ)
This identity links the tangent and secant functions. It can be derived by dividing the first Pythagorean identity by cos²(θ), leading to the relationship between the secant and tangent functions.
cosec²(θ) = 1 + cot²(θ)
This identity relates the cosecant and cotangent functions. It is derived by dividing the first Pythagorean identity by sin²(θ), giving the relationship between cosecant and cotangent.

Trigonometric Identities and Formula

2. Reciprocal Identities

These identities relate the basic trigonometric functions to their reciprocal counterparts:

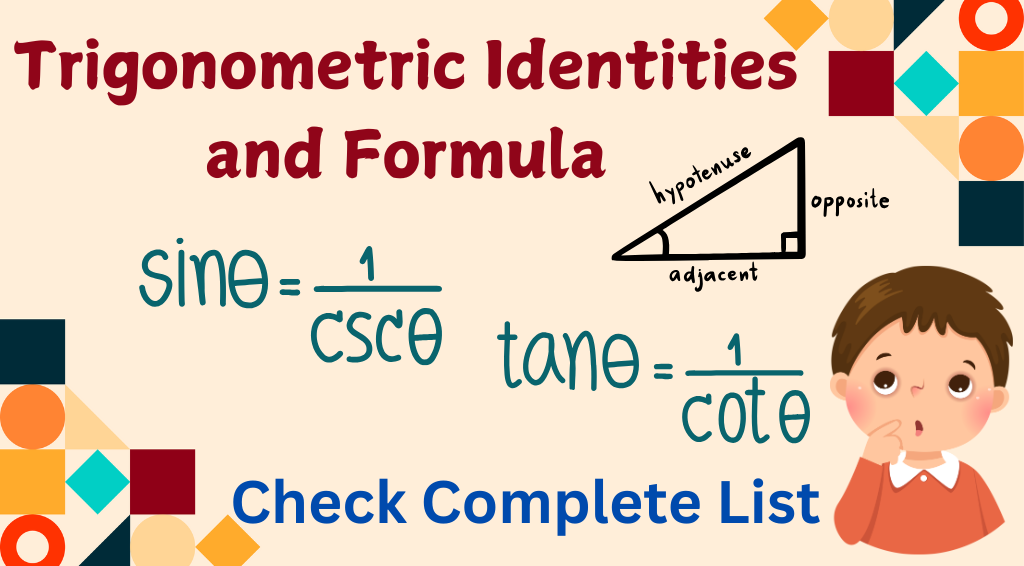
sin x = 1/cosec x
cos x = 1/sec x
tan x = 1/cot x
cot x = 1/tan x
sec x = 1/cos x
cosec x = 1/sin

Trigonometric Identities and Formula

3. Complementary and Supplementary Identities

Complementary angles are two angles whose sum is 90°. The complement of an angle

sin (90°- θ) = cos θ
cos (90°- θ) = sin θ
tan (90°- θ) = cot θ
cosec (90°- θ) = sec θ
sec (90°- θ) = cosec θ
cot (90°- θ) = tan θ

Supplementary angles are two angles whose sum is 180°. The supplement of an angle θ\thetaθ is (180∘−θ)(180^\circ – \theta)(180∘−θ). The trigonometric ratios for supplementary angles are:

sin (180°- θ) = sinθ
cos (180°- θ) = -cos θ
tan (180°- θ) = -tan θ
cosec (180°- θ) = cosec θ
sec (180°- θ)= -sec θ
cot (180°- θ) = -cot θ

4. Even-Odd Identities

These identities explain how trigonometric functions behave under the negation of the angle:

sin⁡(−θ)=−sin⁡(θ)\sin(-\theta) = -\sin(\theta)sin(−θ)=−sin(θ) (Sine is odd)
cos⁡(−θ)=cos⁡(θ)\cos(-\theta) = \cos(\theta)cos(−θ)=cos(θ) (Cosine is even)
tan⁡(−θ)=−tan⁡(θ)\tan(-\theta) = -\tan(\theta)tan(−θ)=−tan(θ) (Tangent is odd)
cot⁡(−θ)=−cot⁡(θ)\cot(-\theta) = -\cot(\theta)cot(−θ)=−cot(θ) (Cotangent is odd)
sec⁡(−θ)=sec⁡(θ)\sec(-\theta) = \sec(\theta)sec(−θ)=sec(θ) (Secant is even)
csc⁡(−θ)=−csc⁡(θ)\csc(-\theta) = -\csc(\theta)csc(−θ)=−csc(θ) (Cosecant is odd)

Trigonometric Identities and Formula

5. Sum and Difference Identities

These identities express the trigonometric functions of the sum or difference of two angles:

sinA+sinB=2[sin((A+B)/2)⋅cos((A−B)/2)]
sinA−sinB=2[cos((A+B)/2)⋅sin((A−B)/2)]
cosA+cosB=2[cos((A+B)/2)⋅cos((A−B)/2)]
cosA−cosB=−2[sin((A+B)/2)⋅sin((A−B)/2)]

Product to Sum Identities: These identities are: sin A⋅

cos B = [sin(A + B) + sin(A − B)]/2 cos A⋅
cos B = [cos(A + B) + cos(A − B)]/2 sin A
sin B = [cos(A − B) − cos(A + B)]/2

Trigonometric Identities and Formula

6. Ratio Trigonometric Identities

Ratio Trigonometric Identities refer to the relationships between the six primary trigonometric functions (sine, cosine, tangent, cosecant, secant, and cotangent) that are derived from the ratios of the sides of a right-angled triangle.

These identities express how each of the six functions is related to one another. The basic ratio trigonometric identities are as follows:

Tan θ = Sin θ/Cos θ
Cot θ = Cos θ/Sin θ

Trigonometric Identities and Formula

7. Trigonometric Identities of Opposite Angles

Trigonometric Identities of Opposite Angles refer to the relationships between trigonometric functions for an angle and its opposite (or negative) counterpart. These identities show how the values of trigonometric functions change when the angle is measured in the opposite direction, typically in the negative direction. They are important for simplifying expressions involving negative angles and understanding the symmetry of trigonometric functions. The key identities for opposite angles are:

Sin (-θ) = – Sin θ
Cos (-θ) = Cos θ
Tan (-θ) = – Tan θ
Cot (-θ) = – Cot θ
Sec (-θ) = Sec θ
Csc (-θ) = -Csc θ

Trigonometric Identities and Formula

8. Trigonometric Identities of Complementary Angles

Trigonometric Identities of Complementary Angles are based on the concept of complementary angles, which are two angles that add up to 90°. These identities describe the relationships between the trigonometric functions of complementary angles (angles that sum up to 90°). They are also known as co-function identities because they connect the trigonometric functions to each other in pairs. The identities for complementary angles are as follows:

Sin (90 – θ) = Cos θ
Cos (90 – θ) = Sin θ
Tan (90 – θ) = Cot θ
Cot ( 90 – θ) = Tan θ
Sec (90 – θ) = Csc θ
Csc (90 – θ) = Sec θ

9. Trigonometric Identities of Supplementary Angles

Two angles are supplementary if their sum is equal to 90 degrees. Similarly, when we can learn here the trigonometric identities for supplementary angles.

sin (180°- θ) = sinθ
cos (180°- θ) = -cos θ
cosec (180°- θ) = cosec θ
sec (180°- θ)= -sec θ
tan (180°- θ) = -tan θ
cot (180°- θ) = -cot θ

10. Sum and Difference of Angles Trigonometric Identities

Sum and Difference of Angles Trigonometric identities are used to find the trigonometric values of the sum or difference of two angles. These identities express the trigonometric functions of sin, cos, and tan in terms of the trigonometric functions of individual angles, making them useful in simplifying expressions or solving trigonometric equations. Below are the key sum and difference identities:

sin(α+β)=sin(α).cos(β)+cos(α).sin(β)
sin(α–β)=sinα.cosβ–cosα.sinβ
cos(α+β)=cosα.cosβ–sinα.sinβ
cos(α–β)=cosα.cosβ+sinα.sinβ
tan⁡(α+β)=tan⁡α+tan⁡β1–tan⁡α.tan⁡β
tan⁡(α–β)=tan⁡α–tan⁡β1+tan⁡α.tan⁡β

Trigonometric Identities and Formula

11. Double Angle Trigonometric Identities

Double Angle Trigonometric Identities are used to express trigonometric functions of double angles (i.e., 2𝜃) in terms of single angles. These identities are derived from the sum of angles identities and are important tools in simplifying trigonometric expressions and solving equations. Below are the commonly used double angle identities:

sin 2θ = 2 sinθ cosθ
cos 2θ = cos2θ – sin2 θ = 2 cos2θ – 1 = 1 – 2sin2 θ
tan 2θ = (2tanθ)/(1 – tan2θ)

Trigonometric Identities and Formula

12. Half Angle Identities

sin (θ/2) = ±√[(1 – cosθ)/2]
cos (θ/2) = ±√(1 + cosθ)/2
tan (θ/2) = ±√[(1 – cosθ)(1 + cosθ)]

Trigonometric Identities and Formula

13. Product-Sum Trigonometric Identities

Product-Sum Trigonometric Identities are used to express the product of two trigonometric functions as a sum or difference. These identities are derived from the sum and difference formulas for trigonometric functions and are useful for simplifying expressions in integrals, solving trigonometric equations, and other applications in mathematics, physics, and engineering.

Sin A + Sin B = 2 Sin(A+B)/2 . Cos(A-B)/2
Cos A + Cos B = 2 Cos(A+B)/2 . Cos(A-B)/2
Sin A – Sin B = 2 Cos(A+B)/2 . Sin(A-B)/2
Cos A – Cos B = -2 Sin(A+B)/2 . Sin(A-B)/2

Trigonometric Identities and Formula

References

Khushi

My name is Khushi, I am a content writer and I provide news related to government jobs and I am from Rajasthan and I only write on this website.