Hyperbola: Definition, Equation, Properties, and Examples

Hyperbola: Definition, Equation, Properties, and Examples

A hyperbola functions is a geometric curve with two symmetrical branches. Explore the derivation of standard and general equations of a hyperbola with solved examples.

A Hyperbola functions is a fascinating geometric figure made up of two symmetrical, curved branches that mirror each other. It is created when a plane slices through both halves of a cone at an angle. Unlike circles or ellipses, a hyperbola is an open curve that stretches infinitely.

In this blog, we will explore the parts, properties, equations, and other aspects of a hyperbola to provide a clear understanding of this unique shape.

What is a Hyperbola?

A hyperbola functions is the set of all points (locus) where the difference between their distances to two fixed points, called foci, remains constant. This difference is calculated by subtracting the distance to the closer focus from the distance to the farther focus.

If P(x,y) is a point on the F and F′ are the two foci, the hyperbola is defined by:

∣PF−PF′∣= 2a

Here,

• PF: The distance from the point P to the first focus F.
• PF′′: The distance from the point P to the second focus F′.
• 2a: A fixed constant that defines the shape and size of the hyperbola. It is directly related to the geometry of the curve and helps determine the distance between the two vertices.

This constant value, 2a, ensures that the maintains its unique property at every point on the curve.

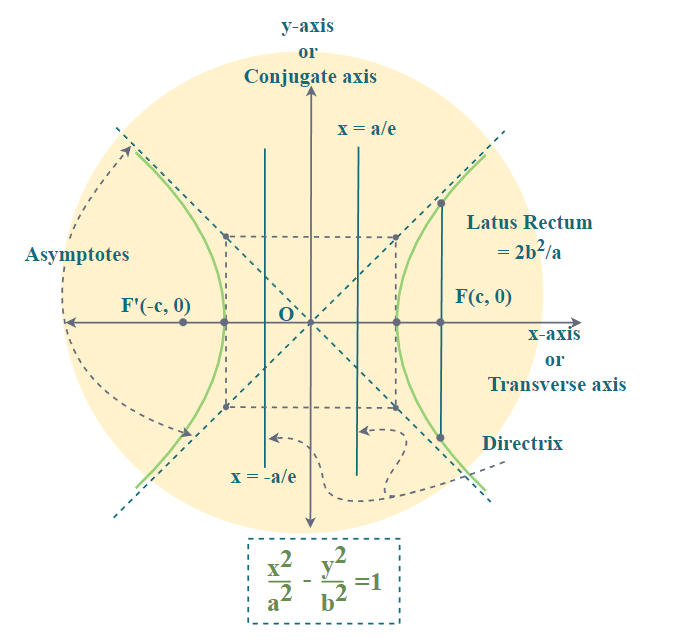
Parts of a Hyperbola

A different parts, which are mentioned as follows:

Properties of Hyperbola

• The difference between distances from any point on the hyperbola to its two foci is constant, i.e. ∣PS−PS′∣=2a
• A hyperbola is rectangular if the transverse and conjugate axes are equal, with eccentricity √2.
• The latus rectum, a line perpendicular to the transverse axis passing through a focus, has length 2b²/a
• Asymptotes are lines passing through the centre that approach the hyperbola but never intersect it.
• The auxiliary circle is centered at the hyperbola’s centre with the transverse axis as its diameter, and its equation is x²+y² =a²
• A conjugate are equal if they have the same latus rectum.

Standard Equations of Hyperbola

The equations of a hyperbola depend on the orientation of its transverse and conjugate axes. The standard forms are as follows:

For a hyperbola with the transverse axis along the X-axis:
x²/a² − y²b² = 1

For a hyperbola with the transverse axis along the Y-axis:
y²/a² − x²b² =1

Generalized Equations of Hyperbola with Center at (h,k)

When the center is at (h, k), the equations adjust as follows:

For the transverse axis along the x-axis:
(x−h)²/ a ²−(y−k)²/b² = 1

For the transverse axis along the y-axis:
(y−k)²/a² −(x−h)²b² =1

Here,

• a²: Denotes the square of the semi-major axis.
• b²: Denotes the square of the semi-minor axis.
• h,k: Represent the coordinates of the hyperbola’s center.

These equations describe the geometric properties of the hyperbola and vary depending on the orientation of its axes.

Eccentricity of Hyperbola

The eccentricity, denoted by e, measures how “stretched” the curve is. It is defined as the ratio of the distance of a point on the from the focus to its perpendicular distance from the directrix.

For the eccentricity is always greater than 1 (e>1e). It can be calculated using the formula:

e= √1+b²a²

Here:

• a is the length of the semi-major axis.
• b is the length of the semi-minor axis.

Latus Rectum of Hyperbola

The latus rectum is a line that passes through one of its foci and is perpendicular to the transverse axis. The endpoints of the latus rectum lie on the hyperbola, and its length is given by:

Length of latus rectum= 2b²/a

These properties are fundamental to understanding the geometry of a hyperbola.

1: Determine the eccentricity of the hyperbola x²/81−y²/49=1.

Solution:
The equation of the hyperbola is  x²/81−y²/49=1.

By comparing with the standard equation x²/a² − y²b² = 1, we get:

a² = 81, b²=49a

a = 9, b = 7 

The formula for the eccentricity is:

e= √1+b²/a²​​

Substituting the values of a and b:

e= √1+ 7²/9²​​

e = √1 + 49/81

e = √ 81 + 49/81

e = √130/81

e =√130/9

Hence, the eccentricity of the given is √130/9

2: If the equation is (x−3)²/36 − (y+5)/16 = 1, find the lengths of the major axis, minor axis, and latus rectum.

Solution:
The equation of the hyperbola is (x−3)²/36 − (y+5)²/ 16 =1

By comparing with the standard equation (x−h)²/a²−(y−k)²/b² =1, we get:

h=3, k=−5, a² =36, b² =16h = 3

Length of the major axis:

2a=2×6=12 units

Length of the minor axis:

2b=2×4=8 units

Length of the latus rectum:

2b²/a=2×16/6=32/6=5.33 units

Hence, the lengths are:

• Major axis: 12 units
• Minor axis: 8 units
• Latus rectum: 5.33 units