Diagonal of Rectangle: Meaning, Properties, Formula, and Examples

Diagonal of rectangle is the line connecting opposite corners. Learn the diagonal of a rectangle properties, and formula to calculate the diagonal length easily.

The Diagonal of a rectangle is a very common concept in geometry that becomes handy in different real-life situations, such as identifying the size of a television. This important mathematical concept helps you in various types of scientific calculations and finding quick solutions.

This article gives an idea of the diagonal of a rectangle, its characteristics, and its formula, along with solved examples for better understanding and applications.

What is the Diagonal of Rectangle?

A rectangle is a two-dimensional geometric shape with four sides. Two opposite sides are equal and parallel, and all the internal angles are 90 degrees.

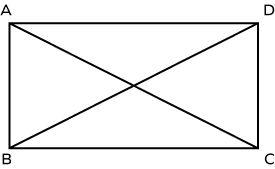
The diagonal of a rectangle refers to the line segment that connects the two opposite vertices of the rectangle. So, a rectangle has two diagonals, which are equal in length.

In the above figure, ABCD is a rectangle, and its two diagonals are AC and BD, which are equal in length.

Diagonal of Rectangle Properties

The diagonal of rectangle have some unique properties, which are discussed below.

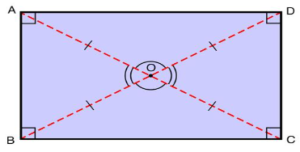
1. The two diagonals of a rectangle bisect each other at the point of intersection.

In the above figure, two diagonals bisect each other at point O, so we can say AO = OC and BO = OD.

2. When the diagonals bisect each other, a pair of acute angles and a pair of obtuse angles are formed at the point of intersection.

In the above figure, angles AOB and COD are acute angles, and angles AOD and BOC are obtuse angles.

3. Diagonals of a rectangle do not meet at a right angle in the center. However, the adjacent angle pairs add up to 180 degrees, which means they are supplementary.

∠AOD + ∠COD = 180 degrees

∠AOB + ∠BOC = 180 degrees

∠AOB + ∠AOD = 180 degrees

∠BOC + ∠COD = 180 degrees

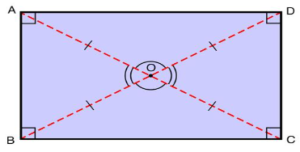
4. The diagonals divide the rectangle into two equal right-angled triangles.

In the above figure, the diagonal AC divides the rectangle into two identical right-angled triangles, ABC and ADC. Similarly, diagonal BD divides the rectangle into two identical right-angled triangles, ABD and BCD

5. The two diagonals of a rectangle divide the rectangle into two pairs of congruent triangles.

In the above figure, the triangles AOB and COD are congruent, and triangles AOD and BOC are congruent.

Formula of Diagonal of Rectangle

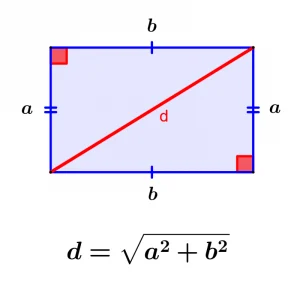
The diagonal of a rectangle divides the rectangle into two right-angled triangles, so it is considered the hypotenuse of these triangles. The formula of diagonal of rectangle can be obtained using the Pythagoras theorem.

Let us consider a rectangle of length “b” and width “a”. Let the length of the diagonal be “d”.

Applying the Pythagoras theorem, we can write,

d² = a² + b²

Taking square root on both sides,

d = √( a² + b²)

Thus, the diagonal of a rectangle formula is:

d = √( a² + b²)

Where

d = diagonal of rectangle
b = length of the rectangle
a = width of the rectangle

How to find the Diagonal of Rectangle Solved Examples

1. Using the diagonal of the rectangle formula, find the diagonal of a rectangular park whose dimensions are 30 ft and 40 ft.

Solution:

As per the formula of diagonal, if the length and width of a rectangle are l and w, then the diagonal can be calculated as d = √( l2 + w2)

Here, l = 40 and w = 30

So, diagonal d = √(40)2+(30)2 = √2500 = 50

Answer: The diagonal of the park is 50 ft.

2. If a television screen has a length of 30 inches and a width of 20 inches. What is the size of the television?

Solution:

The size of the television is the length of its diagonal.

The length of the television, l = 30

The width of the television, w = 20

Using the diagonal of a rectangle formula,

d = √( l² + w²)

d = √( 30² + 20²)

= √1300

= 36.05

Answer: The size of the television is 36.05 inches.

3. If a rectangular table has a width of 5 inches with a diagonal of 13 inches. What is the length of the table?

Solution:

The width of the table, w = 5 inches.

The diagonal of the table, d = 13 inches.

Using the diagonal of rectangle formula,

d = √( l² + w²)

or, 13²= (l² + 5²)

or, l² + 25 = 169

or, l2 = 169 -25 = 144

or, l = 12

Answer: The length of the table is 12 inches.

4. If the area of a rectangular lawn is 480 square meters and the length is 30 meters, what is the length of the diagonal of the lawn?

Solution:

The area of a rectangle is A = Length (l) X Width (w)

The length of the lawn, l = 30 and the area, A = 480

Therefore, 450 = 30 x w

or, w = 450/30 = 16

So, the width of the lawn is 16 meters.

Now, using the diagonal of rectangle formula, we can write

Diagonal d = √( l2 + w2)

= √( 302 + 162)

= √900 + 256

= √1156

= 34

Answer: the diagonal of the lawn is 34 meters.

Reference

Khushi

My name is Khushi, I am a content writer and I provide news related to government jobs and I am from Rajasthan and I only write on this website.